Time Dilation

A derivation of time dilation from Modern Physics 3rd Edition Section 1.5.

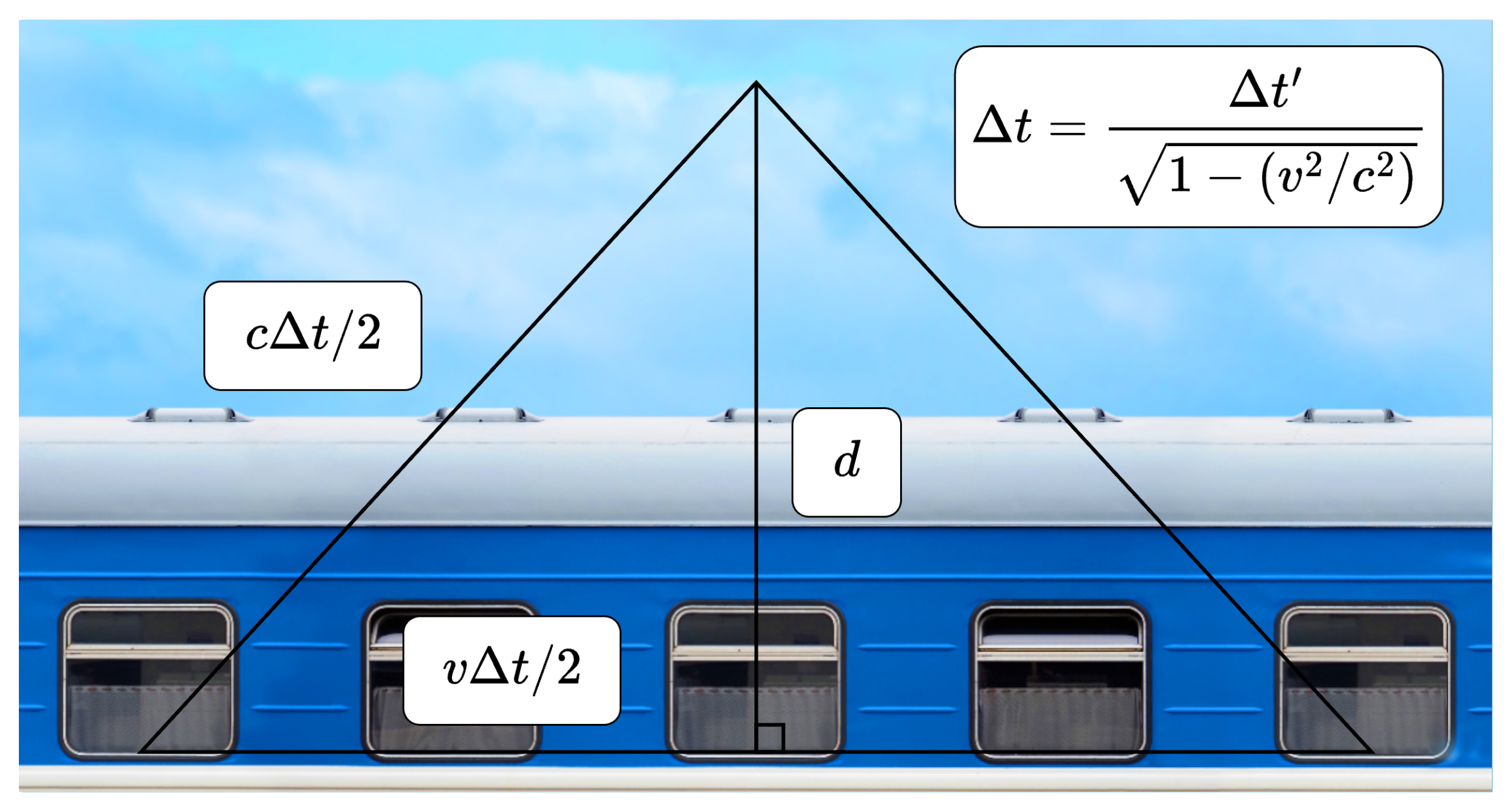
Experimental Setup

• A moving observer on a train moving with speed $v$.
• A stationary observer that is not on the train.
• The moving observer reflects a light beam off of a mirror moving with the train that is $d$ units away.
• The stationary observer also observes the reflected light beam, but with the interaction of the train’s velocity.

We will be using the two postulates of special relativity;

• laws of physics are invariant in all inertial frames of reference (principle of relativity).
• all observers see light travel at the same speed, $c$.

Although the light beam’s origin will be the same for both observers, due to the principle of relativity, we can “shift” the stationary observers origin to form a right triangle (which is helpful for the math).

Solution

Our goal will be to relate a change in time as measured in $\Delta t$ to the change in time as observed on the moving train $\Delta t’$. First, we can compute $\Delta t’$ using $v = \Delta x / \Delta t$:

\[\begin{equation} \Delta t' = \frac{2d}{c} \end{equation}\]

In the stationary frame of reference, we see the moving observer move a distance of $v \Delta t / 2$ and we see the light travel diagonally a distance of $c \Delta t / 2$ for a total vertical height of $d$. We can relate these quantities using the pythagorean theorem for this right triangle:

\[\begin{flalign*} (c\Delta t / 2)^2 &= d^2 + (v \Delta t / 2)^2 \\ c^2 \Delta t^2 / 4 - v^2 \Delta t^2 / 4 &= d^2 \\ \Delta t^2 \cdot (\frac{c^2 - v^2}{4}) &= d^2 \\ \Delta t^2 &= \frac{4d^2}{c^2 - v^2} \\ \Delta t &= \frac{2d}{\sqrt{c^2 - v^2}} \\ &= \frac{2d}{\sqrt{c^2(1 - v^2 / c^2)}} \\ &= \frac{2d}{c \sqrt{(1 - v^2 / c^2)}} \\ &= \frac{2d}{c}\cdot \frac{1}{\sqrt{(1 - v^2 / c^2)}} \\ & = \Delta t' \cdot \frac{1}{\sqrt{(1 - v^2 / c^2)}} \\ & = \gamma \Delta t' \end{flalign*}\]

Since $\gamma >= 1$, we know that $\Delta t > \Delta t’$ and that the stationary observer’s time has dilated (i.e. increased) when viewed from the moving observer’s train car.

Interpretation

Another interpretation is that since the distance the light travels in the stationary frame is strictly greater than the moving frame (hypotenuse vs. leg of a right triangle):

\[\begin{flalign*} c\Delta t / 2 &> d \\ c\Delta t &> 2d \end{flalign*}\]

and the speed of light $c$ has to be the same in each reference then the time in the stationary frame has to be longer to account for this extra distance. For example, for $\Delta t$ to double with respect to $\Delta t’$, the train would need a velocity of:

\[\begin{flalign*} \gamma &= 2 \\ \frac{1}{\sqrt{(1 - v^2 / c^2)}} &= 2 \\ \frac{1}{(1 - v^2 / c^2)} &= 4 \\ \frac{1}{4} &= 1 - v^2 / c^2 \\ v^2 / c^2 &= 3/4 \\ v &= \frac{\sqrt{3}}{2} c \\ &=0.87 \cdot c \end{flalign*}\]

but both observers will still see light moving at speed, $c$!

Demo

Here is a short demo using neopixel strips and a raspberry pi pico controller. The red light represents the moving observer and the green light represents the (shifted) stationary observer: